ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
ਦਿੱਖ
-
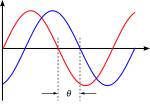
ਇੱਕ ਸਾਈਨੁਸੋਆਇਡਲ ਵਕਰ, ਜਿਸਦਾ ਉੱਚਤਮ ਐਂਪਲੀਟਿਊਡ (1) ਹੈ।
-
ਫੇਜ਼ ਸ਼ਿਫਟ θ ਦਾ ਚਿੱਤ੍ਰਣ।
| ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ |
|---|
| ਨਿਰੰਤਰ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ |
| ਫੋਰੀਅਰ ਸੀਰੀਜ਼ |
| ਅਨਿਰੰਤਰ-ਸਮਾਂ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ |
| ਅਨਿਰੰਤਰ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ |
| ਕਿਸੇ ਛੱਲੇ ਉੱਪਰ ਅਨਿਰੰਤਰ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ |
| ਫੋਰੀਅਰ ਵਿਸ਼ਲੇਸ਼ਣ |
| ਸਬੰਧਤ ਪਰਿਵਰਤਨ |
ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ ਵਕਤ ਦੇ ਕਿਸੇ ਫੰਕਸ਼ਨ (ਕਿਸੇ ਸਿਗਨਲ) ਨੂੰ ਅਜਿਹੀਆਂ ਫਰੀਕੁਐਂਸੀਆਂ ਵਿੱਚ ਤੋੜ ਦਿੰਦਾ ਹੈ ਜੋ ਇਸਨੂੰ ਉਸੇ ਤਰੀਕੇ ਨਸਾਲ ਬਣਾ ਦਿੰਦੀਆਂ ਹਨ, ਜਿਵੇਂ ਕੋਈ ਸੰਗੀਤਕ ਤਾਰ ਨੂੰ ਇਸਦੇ ਰਚਣਹਾਰੇ ਨੋਟਾਂ ਦੇ ਐਂਪਲੀਟਿਊਡ (ਜਾਂ ਉੱਚੇਪਣ) ਦੇ ਤੌਰ 'ਤੇ ਦਰਸਾਇਆ ਜਾ ਸਕਦਾ ਹੈ।
ਪਰਿਭਾਸ਼ਾ
[ਸੋਧੋ]ਇਤਿਹਾਸ
[ਸੋਧੋ]ਜਾਣ-ਪਛਾਣ
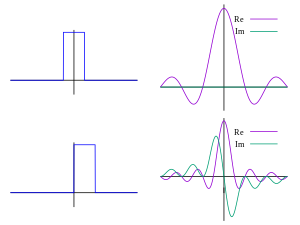
[ਸੋਧੋ]ਉਦਾਹਰਨ
[ਸੋਧੋ]ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ ਦੀਆਂ ਵਿਸ਼ੇਸ਼ਤਾਵਾਂ
[ਸੋਧੋ]ਅਧਾਰ ਵਿਸ਼ੇਸ਼ਤਾਵਾਂ
[ਸੋਧੋ]ਉਲਟਾਤਮਿਕਤਾ ਅਤੇ ਆਵਰਤਾਮਿਕਤਾ
[ਸੋਧੋ]ਇਕਾਈਆਂ ਅਤੇ ਦੋਹਰਾਤਮਿਕਤਾ
[ਸੋਧੋ]ਇੱਕਸਾਰ ਨਿਰੰਤ੍ਰਤਾ ਅਤੇ ਰੀਮਾੱਨ-ਲੈਬਸਗਿਉ ਲੈੱਮਾ
[ਸੋਧੋ]ਪਲਾਚਿਰਿਲ ਥਿਊਰਮ ਅਤੇ ਪਾਰਸਵਲ ਦੀ ਥਿਊਰਮ
[ਸੋਧੋ]ਪੋਆਇਸ਼ਨ ਜੋੜ ਫਾਰਮੂਲਾ
[ਸੋਧੋ]ਡਿੱਫ੍ਰੈਂਟੀਏਸ਼ਨ
[ਸੋਧੋ]ਕਨਵੋਲਿਊਸ਼ਨ ਥਿਊਰਮ
[ਸੋਧੋ]ਆਰਪਾਰ-ਸਹਿਸਬੰਧ ਥਿਊਰਮ
[ਸੋਧੋ]ਆਈਗਬ-ਫੰਕਸ਼ਨ
[ਸੋਧੋ]ਹੇਜ਼ਨਬਰਗ ਗਰੁੱਪ ਨਾਲ ਸਬੰਧ
[ਸੋਧੋ]ਕੰਪਲੈਕਸ ਡੋਮੇਨ
[ਸੋਧੋ]ਲਾਪਲੇਸ ਪਰਿਵਰਤਨ
[ਸੋਧੋ]ਪਲਟੀ
[ਸੋਧੋ]ਯੁਕਿਲਡਨ ਸਪੇਸ ਉੱਤੇ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
[ਸੋਧੋ]ਅਨਸਰਟਨੀ ਸਿਧਾਂਤ
[ਸੋਧੋ]ਸਾਈਨ ਅਤੇ ਕੋਜ਼ਾਈਨ ਪਰਿਵਰਤਨ
[ਸੋਧੋ]ਗੋਲ ਹਾਰਮੋਨਿਕਾਂ
[ਸੋਧੋ]ਮਨਾਹੀ ਸਮੱਸਿਆਵਾਂ
[ਸੋਧੋ]ਫੰਕਸ਼ਨ ਸਪੇਸ ਉੱਤੇ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
[ਸੋਧੋ]Lp ਸਪੇਸਾਂ ਉੱਤੇ
[ਸੋਧੋ]ਛੇੜੀਆਂ ਹੋਈਆਂ ਵਿਸਥਾਰ-ਵੰਡਾਂ
[ਸੋਧੋ]ਸਰਵ-ਸਧਾਰੀਕਰਨਾਂ
[ਸੋਧੋ]ਫੋਰੀਅਰ-ਸਟਿਲਟਜਸ ਪਰਿਵਰਤਨ
[ਸੋਧੋ]ਸਥਾਨਿਕ ਤੌਰ 'ਤੇ ਸੁੰਗੜਿਆ ਅਬੇਲੀਅਨ ਗਰੁੱਪ
[ਸੋਧੋ]ਗਲਫਾਂਡ ਪਰਿਵਰਤਨ
[ਸੋਧੋ]ਸੁੰਗੜੇ ਗੈਰ-ਅਬੇਲੀਅਨ ਗਰੁੱਪ
[ਸੋਧੋ]ਬਦਲ
[ਸੋਧੋ]ਉਪਯੋਗ
[ਸੋਧੋ]ਡਿੱਫ੍ਰੈਂਸ਼ੀਅਲ ਇਕੁਏਸ਼ਨਾਂ ਦਾ ਵਿਸ਼ੇਲੇਸ਼ਣ
[ਸੋਧੋ]ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ ਸਪੈਕਟ੍ਰੋਸਕੋਪੀ
[ਸੋਧੋ]ਕੁਆਂਟਮ ਮਕੈਨਿਕਸ
[ਸੋਧੋ]ਸੰਕੇਤ ਵਿਕਾਸ
[ਸੋਧੋ]ਹੋਰ ਧਾਰਨਾਵਾਂ
[ਸੋਧੋ]ਹੋਰ ਪ੍ਰੰਪ੍ਰਾਵਾਂ
[ਸੋਧੋ]ਹਿਸਾਬ ਵਿਧੀਆਂ
[ਸੋਧੋ]ਬੰਦ-ਅਕਾਰ ਫੰਕਸ਼ਨਾਂ ਦੀ ਸੰਖਿਅਨ ਇੰਟੀਗ੍ਰੇਸ਼ਨ
[ਸੋਧੋ]ਵਿਵਸਥਿਤ ਜੋੜਿਆਂ ਦੀ ਇੱਕ ਲੜੀ ਦੀ ਸੰਖਿਅਕ ਇੰਟੀਗ੍ਰੇਸ਼ਨ
[ਸੋਧੋ]ਅਨਿਰੰਤਰ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ ਅਤੇ ਤੇਜ਼ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
[ਸੋਧੋ]ਮਹੱਤਵਪੂਰਨ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨਾਂ ਦੀ ਸਾਰਣੀ
[ਸੋਧੋ]ਫੰਕਸ਼ਨਲ ਸਬੰਧਾਂ
[ਸੋਧੋ]ਵਰਗ-ਇੰਟੀਗ੍ਰੇਟਯੋਗ ਫੰਕਸ਼ਨ
[ਸੋਧੋ]ਵਿਸਥਾਰ-ਵੰਡਾਂ
[ਸੋਧੋ]ਦੋ-ਅਯਾਮੀ ਫੰਕਸ਼ਨ
[ਸੋਧੋ]ਸਰਵ-ਸਧਾਰਬ n-ਅਯਾਮੀ ਫੰਕਸ਼ਨਾਂ ਵਾਸਤੇ ਸੂਤਰ
[ਸੋਧੋ]ਇਹ ਵੀ ਦੇਖੋ
[ਸੋਧੋ]- ਐਨਾਲੌਗ ਸੰਕੇਤ ਵਿਕਾਸ
- ਬੀਵਰਜ਼-ਲਿਪਸਨ ਸਟਰਿਪ
- ਅਨਿਰੰਤਰ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
- ਅਨਿਰੰਤਰ-ਸਮਾਂ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
- ਤੇਜ਼ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
- ਫੋਰੀਅਰ ਇੰਟਗ੍ਰਲ ਓਪਰੇਟਰ
- ਫੋਰੀਅਰ ਇਨਵਰਸ਼ਨ ਥਿਓਰਮ
- ਫੋਰੀਅਰ ਮਲਟੀਪਲਾਇਰ
- ਫੋਰੀਅਰ ਸੀਰੀਜ਼
- ਫੋਰੀਅਰ ਸਾਈਨ ਪਰਿਵਰਤਨ
- ਫੋਰੀਅਰ-ਡੈਲੀਗਨੇ ਪਰਿਵਰਤਨ
- ਫੋਰੀਅਰ-ਮੁਕਾਇ ਪਰਿਵਰਤਨ
- ਭਿੰਨਾਤਮਿਕ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
- ਅਸਿੱਧਾ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
- ਇੰਟਗ੍ਰਲ ਪਰਿਵਰਤਨ
- ਲਾਪਲੇਸ ਪਰਿਵਰਤਨ
- ਰੇਖਿਕ ਕਾਨੋਨੀਕਲ ਪਰਿਵਰਤਨ
- ਮਿਲਿੱਨ ਪਰਿਵਰਤਨ
- ਬਹੁਅਯਾਮੀ ਪਰਿਵਰਤਨ
- NGC 4622, ਖਾਸਤੌਰ 'ਤੇ ਤਸਵੀਰ NGC 4622 ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ m = 2.
- ਅਲਪ-ਕਾਲੀਨ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
- ਸਪੇਸ-ਟਾਈਮ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
- ਸਪੈਕਟ੍ਰਲ ਘਣਤਾ
- ਚਿੰਨਾਤਮਿਕ ਇੰਟੀਗ੍ਰੇਸ਼ਨ
- ਸਮਾਂ ਖਿੱਚ ਡਿਸਪਰਸਿਵ ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ
- ਪਰਿਵਰਤਨ (ਗਣਿਤ)
ਟਿੱਪਣੀਆਂ
[ਸੋਧੋ]ਨੋਟਸ
[ਸੋਧੋ]ਹਵਾਲੇ
[ਸੋਧੋ]- Boashash, B., ed. (2003), Time-Frequency Signal Analysis and Processing: A Comprehensive Reference, Oxford: Elsevier Science, ISBN 0-08-044335-4
- Bochner S., Chandrasekharan K. (1949), Fourier Transforms, Princeton University Press
- Bracewell, R. N. (2000), The Fourier Transform and Its Applications (3rd ed.), Boston: McGraw-Hill, ISBN 0-07-116043-4.
- Campbell, George; Foster, Ronald (1948), Fourier Integrals for Practical Applications, New York: D. Van Nostrand Company, Inc..
- Condon, E. U. (1937), "Immersion of the Fourier transform in a continuous group of functional transformations", Proc. Natl. Acad. Sci. USA, 23: 158–164.
- Duoandikoetxea, Javier (2001), Fourier Analysis, American Mathematical Society, ISBN 0-8218-2172-5.
- Dym, H; McKean, H (1985), Fourier Series and Integrals, Academic Press, ISBN 978-0-12-226451-1.
- Erdélyi, Arthur, ed. (1954), Tables of Integral Transforms, vol. 1, New Your: McGraw-Hill
- Gerald Folland (1989), Harmonic analysis in phase space, Princeton University Press
- Fourier, J. B. Joseph (1822), Théorie Analytique de la Chaleur, Paris: Chez Firmin Didot, père et fils
- Fourier, J. B. Joseph; Freeman, Alexander, translator (1878), The Analytical Theory of Heat, The University Press
{{citation}}:|first2=has generic name (help)CS1 maint: multiple names: authors list (link) - Champeney, D.C. (1987), A Handbook of Fourier Theorems, Cambridge University Press
- de Groot, Sybren R.; Mazur, Peter (1984), Non-Equilibrium Thermodynamics (2nd ed.), New York: Dover
- Marín Antuña, José (1990), Teoría de funciones de variable compleja (2nd ed.), Havana: Editorial Pueblo y Educación
- Chatfield, Chris (2004), The Analysis of Time Series: An Introduction, Texts in Statistical Science (6th ed.), London: Chapman & Hall/CRC
- Feller, William (1971), An Introduction to Probability Theory and Its Applications. Vol. II. (Second ed.), New York: John Wiley & Sons, MR 0270403.
- Wiener, Norbert (1949), Extrapolation, Interpolation, and Smoothing of Stationary Time Series With Engineering Applications, Cambridge, Mass.: Technology Press and John Wiley & Sons and Chapman & Hall
- Kirillov, Alexandre; Gvichiani, Alexei (1982), Théorèmes et problèmes d'analyse fonctionnelle, Djilali Embarek, translator, Moscow: Mir
- Kolmogórov, Andréi Nikolaevich; Fomín, Serguei Vasílievich (1978), Elementos de la teoría de funciones y del análisis functional, Carlos Vega, translator (3rd ed.), Moscow: Mir
- Guelfand, Israel Moiseevich; Vilenkin, N.Y. (1967), Les distributions tome 4: applications de l'analyse harmonique, G. Rideau, translator, Paris: Dunod
- Widder, David Vernon; Wiener, Norbert (August 1938), "Remarks on the Classical Inversion Formula for the Laplace Integral", Bulletin of the American Mathematical Society, 44: 573–575, doi:10.1090/s0002-9904-1938-06812-7
- Clozel, Laurent; Delorme, Patrice (1985), "Sur le théorème de Paley-Wiener invariant pour les groupes de Lie réductifs réels", C. R. Acad. Sci. Paris, série I, 300: 331–333
- Paley, R.E.A.C.; Wiener, Norbert (1934), Fourier Transforms in the Complex Domain, American Mathematical Society Colloquium Publications, Providence, Rhode Island: American Mathematical Society
- Grafakos, Loukas (2004), Classical and Modern Fourier Analysis, Prentice-Hall, ISBN 0-13-035399-X.
- Grafakos, Loukas; Teschl, Gerald (2013), "On Fourier transforms of radial functions and distributions", J. Fourier Anal. Appl., 19: 167–179, doi:10.1007/s00041-012-9242-5.
- Greiner, W.; Reinhardt, J. (1996), Field Quantization, Springer Publishing, ISBN 3-540-59179-6
- Hewitt, Edwin; Ross, Kenneth A. (1970), Abstract harmonic analysis. Vol. II: Structure and analysis for compact groups. Analysis on locally compact Abelian groups, Die Grundlehren der mathematischen Wissenschaften, Band 152, Berlin, New York: Springer-Verlag, MR 0262773.
- Hörmander, L. (1976), Linear Partial Differential Operators, Volume 1, Springer-Verlag, ISBN 978-3-540-00662-6.
- James, J.F. (2011), A Student's Guide to Fourier Transforms (3rd ed.), New York: Cambridge University Press, ISBN 978-0-521-17683-5.
- Kaiser, Gerald (1994), A Friendly Guide to Wavelets, Birkhäuser, ISBN 0-8176-3711-7
- Kammler, David (2000), A First Course in Fourier Analysis, Prentice Hall, ISBN 0-13-578782-3
- Katznelson, Yitzhak (1976), An introduction to Harmonic Analysis, Dover, ISBN 0-486-63331-4
- Knapp, Anthony W. (2001), Representation Theory of Semisimple Groups: An Overview Based on Examples, Princeton University Press, ISBN 978-0-691-09089-4
- Müller, Meinard (2015), The Fourier Transform in a Nutshell. (PDF), In Fundamentals of Music Processing, Section 2.1, pages 40-56: Springer, doi:10.1007/978-3-319-21945-5, ISBN 978-3-319-21944-8, archived from the original (PDF) on 2016-04-08, retrieved 2016-06-26
{{citation}}: External link in|location= - Pinsky, Mark (2002), Introduction to Fourier Analysis and Wavelets, Brooks/Cole, ISBN 0-534-37660-6[permanent dead link]
- Polyanin, A. D.; Manzhirov, A. V. (1998), Handbook of Integral Equations, Boca Raton: CRC Press, ISBN 0-8493-2876-4.
- Rudin, Walter (1987), Real and Complex Analysis (Third ed.), Singapore: McGraw Hill, ISBN 0-07-100276-6.
- Rahman, Matiur (2011), Applications of Fourier Transforms to Generalized Functions, WIT Press, ISBN 1845645642.
- Stein, Elias; Shakarchi, Rami (2003), Fourier Analysis: An introduction, Princeton University Press, ISBN 0-691-11384-X.
- Stein, Elias; Weiss, Guido (1971), Introduction to Fourier Analysis on Euclidean Spaces, Princeton, N.J.: Princeton University Press, ISBN 978-0-691-08078-9.
- Taneja, HC (2008), "Chapter 18: Fourier integrals and Fourier transforms", Advanced Engineering Mathematics:, Volume 2, New Delhi, India: I. K. International Pvt Ltd, ISBN 8189866567.
- Titchmarsh, E (1948), Introduction to the theory of Fourier integrals (2nd ed.), Oxford University: Clarendon Press (published 1986), ISBN 978-0-8284-0324-5.
- Vretblad, Anders (2000), Fourier Analysis and its Applications, Graduate Texts in Mathematics, vol. 223, New York: Springer Publishing, ISBN 0-387-00836-5
- Wilson, R. G. (1995), Fourier Series and Optical Transform Techniques in Contemporary Optics, New York: Wiley, ISBN 0-471-30357-7.
- Yosida, K. (1968), Functional Analysis, Springer-Verlag, ISBN 3-540-58654-7.
ਬਾਹਰੀ ਲਿੰਕ
[ਸੋਧੋ]- Weisstein, Eric W., "ਫੋਰੀਅਰ ਪਰਿਵਰਤਨ" from MathWorld.
ਸ਼੍ਰੇਣੀਆਂ:
- Pages using div col with unknown parameters
- CS1 errors: generic name
- CS1 maint: multiple names: authors list
- CS1 errors: external links
- CS1 maint: location
- Articles with dead external links from ਜਨਵਰੀ 2023
- Articles with BNF identifiers
- Pages with authority control identifiers needing attention
- Articles with BNFdata identifiers
- Articles with GND identifiers
- Articles with J9U identifiers
- Articles with NDL identifiers
- Articles with NKC identifiers
- ਫੋਰੀਅਰ ਵਿਸ਼ਲੇਸ਼ਣ
- ਇੰਟਗ੍ਰਲ ਪਰਿਵਰਤਨ
- ਯੂਨਾਇਟਰੀ ਓਪਰੇਟਰ
- ਜੋਜ਼ਫ ਫੋਰੀਅਰ
- ਗਣਿਤਿਕ ਭੌਤਿਕ ਵਿਗਿਆਨ